Question

Graph the exponential function g(x)=(3/5) ^x To graph the function plot the points on the graph with the x values -2 , -1,0,1 and 2

Could somebody tell me what the points are to graph this and how to get the answer?

Answer 1

so you plug in the numbers you were given (-2, -1, 0, 1, 2) into the x value one at a time and you will get the other point for that pair so (3/5)^-2 and whatever number you get is the 2nd number to that ordered pair

Answer 2

The graph of
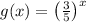`\( g(x) = \left((3)/(5)\right)^x \)` shows a decreasing exponential function. Red points at x = -2, -1, 0, 1, and 2 highlight specific values. As x increases, the function rapidly approaches zero, demonstrating its exponential decay.

The graph illustrates the exponential function
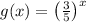`\( g(x) = \left((3)/(5)\right)^x \)`. The red points represent specific values of the function for x = -2, -1, 0, 1, and 2. As x increases, the function rapidly approaches zero, showcasing the decay characteristic of exponential functions.

The curve smoothly traces the continuous behavior of the function between the plotted points. The base
`\((3)/(5)\)` results in a decreasing curve, emphasizing the diminishing trend of the function as x increases. The graph provides a visual representation of how the function's values change exponentially with varying x.