Answer:
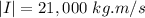
Correct answer: Option E
Step-by-step explanation:
Impulse and Momentum
The Impulse is defined as the change of momentum of an object in a certain interval. The formula is:
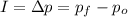
Where pf and po are the final and initial momentums respectively. Knowing that for m= mass of the object and v=velocity (or speed in the scalar form)
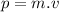
Thus
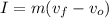
Mr. Hogan's 3000 kg truck is initially at 36 m/s and later slows down to 29 m/s. We compute the impulse as follows

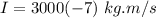

We are asked for the magnitude, thus
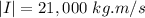
Correct Answer: Option E