Question

HELP ASAP

Use the data below.

30, 24, 34, 37, 50, 32, 32, 35, 39

Part A: Identify the minimum, lower quartile, median, upper quartile, maximum and interquartile range.
Minimum:
Lower Quartile:
Median:
Upper Quartile:
Maximum:
Interquartile Range:
Part B: Is their a outlier? Explain

Answer 1

Explanation:

Arranging the data in ascending order we have :

24 , 30 , 32 , 32 , 34 , 35 , 37 , 39 , 50

(i) The minimum value is therefore 24

(ii) To find the lower quartile , we will need to find the median , that is the number in the middle , the number in the middle is 34 , therefore , the median is 34. Since we are dealing with odd data , that is the total number of data given is 9 , which is an odd number , we will not include the median in the lower quartile , therefore , the lower half of the data are :

24 , 30 , 32 , 32

To find the lower quartile , we will find the median of the lower half.

Median of the lower half =
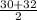`(30+32)/(2)` = 31

Therefore , Lower quartile = 31

(iii) Median = 34

(iv) To find the upper quartile , we will write out the upper half , which are :

35 , 37 , 39 , 50

We will then find the median of the upper half , which will be 37 + 39 / 2

= 76/2

=38

Therefore , Upper quartile = 38

(iv) To find the maximum , we will identify the highest number , therefore , the maximum = 50

(v) Interquartile range = upper quartile - lower quartile

Interquartile range = 38 - 31

Interquartile range = 7

(B) outlier is a data point that differs significantly from other observations

minimum = 24

maximum = 50

median = 34

lower quartile = 31

upper quartile = 38

interquartile range = 7

To find the outliner , we will follow the following steps

(i) multiply the interquartile range by 1.5 = 7 x 1.5 = 10.5

(ii) add it the upper quartile = 10.5 + 38 = 48 .5

(ii) subtract it from the lower quartile = 31 - 10 . 5 = 20 .5

This means that the boundary of the outliner are 20.5 and 48 . 5

The only number that falls outside this is 50

Therefore , there is an outliner