65 adult tickets and 24 children tickets were sold
Solution:
Let "a" be the number of adult tickets sold
Let "c" be the number of children tickets sold
Cost of 1 adult ticket = $ 4.10
Cost of 1 children ticket = $ 2.70
Last Thursday, a total of $331.30 was collected from 89 movie watchers
Number of movie watchers = 89
number of adult tickets sold + number of children tickets sold = 89
a + c = 89
c = 89 - a ----------- eqn 1
They collected a total of $ 331.30
Therefore, we frame a equation as:
number of adult tickets sold x Cost of 1 adult ticket + number of children tickets sold x Cost of 1 children ticket = 331.30
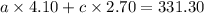
4.1a + 2.7c = 331.3 ------ eqn 2
Substitute eqn 1 in eqn 2
4.1a + 2.7(89 - a) = 331.3
4.1a + 240.3 - 2.7a = 331.3
Combine the like terms
1.4a = 331.3 - 240.3
1.4a = 91
a = 65
Substitute a = 65 in eqn 1
c = 89 - 65
c = 24
Thus 65 adult tickets and 24 children tickets were sold