Question

One boat traveling 15 mph goes 36 miles downstream in the same amount of time that the other boat going 20 mph goes 34 miles upstream. How fast is the current in mph? Enter only the numerical result.

Answer 1

Speed of current = 3

Explanation:

Let the speed of current be 'x'.

Given:

Speed of first boat in still water (s) = 15 mph

Distance traveled by the first boat (d) = 36 miles

Downstream speed =
`s+x`

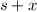
Speed of the second boat in still water (S) = 20 mph

Distance traveled by the (D) = 34 miles

Upstream speed =
`S-x`

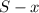
Now, as per question, time for downstream of first boat is equal to time for upstream of second boat.

So, Time taken by first boat downstream is given as:

`Time=(Distance)/(Downstream\ speed)\\\\Time=(d)/(s+x)\\\\Time = (36)/(15+x)-----1`

Time taken by second boat upstream is given as:

`Time=(Distance)/(Upstream\ speed)\\\\Time=(D)/(S-x)\\\\Time = (34)/(20-x)-----2`

Since, time taken is same. Therefore, equating (1) and (2), we get

`(36)/(15+x)=(34)/(20-x)`

Using cross multiplication, we get:

`36(20-x)=34(15+x)\\\\720-36x=510+34x\\\\720-510=34x+36x\\\\210=70x\\\\x=(210)/(70)\\\\x=3\ mph`

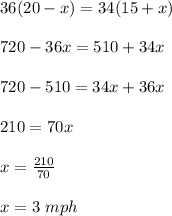
Therefore, the speed of the current is 3 mph.