Question

Carbon dioxide (CO2) is compressed in a piston-cylinder assembly from p1 = 0.7 bar, T1 = 320 K to p2 = 11 bar. The initial volume is 0.262 m3 . The process is described by pV1.25 = constant.

Assuming ideal gas behavior and neglecting kinetic and potential energy effects, determine the work and heat transfer for the process, each in kJ, for (a) constant specific heats evaluated at 300K, and (b) data from Table A- 23.

Compare the results and discuss.

Answer 1

`W_(12)=-53.9056KJ`

Part A:

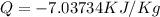`Q=-7.03734 KJ/Kg` (-ve sign shows heat is getting out)

Part B:

`Q=1.5265KJ/Kg` (Heat getting in)

The value of Q at constant specific heat is approximately 361% in difference with variable specific heat and at constant specific heat Q has opposite direction (going in) than Q which is calculated in Part B from table A-23. So taking constant specific heat is not a good idea and is questionable.

Step-by-step explanation:

Assumptions:

1. Gas is ideal
2. System is closed system.
3. K.E and P.E is neglected
4. Process is polytropic

Since Process is polytropic so
`W_(12) =(P_(2)V_(2)-P_(1)V_(1))/(1-n)`

Where n=1.25

Since Process is polytropic :

`(V_(2))/(V_(1))=((P_(1))/(P_(2)))^{(1)/(1.25)} \\V_(2)= ((P_(1))/(P_(2)))^{(1)/(1.25)} *V_(1)`

`V_(2)= ((0.7)/(11))^{(1)/(1.25)} *0.262\\V_(2)=0.028924 m^3`

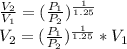
Now,
`W_(12) =(P_(2)V_(2)-P_(1)V_(1))/(1-n)`

`W_(12) =(11*0.028924-0.7*0.262)/(1-1.25)((10^(5)N/m^2)/(1 bar))((1  KJ)/(10^(3)Nm))`

`W_(12)=-53.9056KJ`

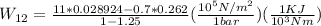
We will now calculate mass (m) and Temperature T_2.

`m=(P_(1)V_(1))/(RT_(1))\\ m=(0.7*0.262)/((8.314KJ)/(44.01Kg.K)*320)((10^(5)N/m^2)/(1 bar))((1  KJ)/(10^(3)Nm))\\m=0.30338Kg`

`T_(2) =(P_(2)V_(2))/(Rm)\\ m=(11*0.028924)/((8.314KJ)/(44.01Kg.K)*0.30338)((10^(5)N/m^2)/(1 bar))((1  KJ)/(10^(3)Nm))\\T_(2) =555.14K`

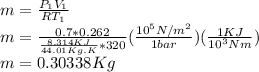
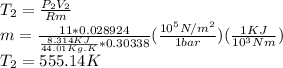
Part A:

According to energy balance::

`Q=mc_(v)(T_(2)-T_(1))+W_(12)`

From A-20, C_v for Carbon dioxide at 300 K is 0.657 KJ/Kg.k

`Q=0.30338*0.657(555.14-320)+(-53.9056)`

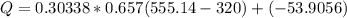
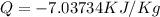`Q=-7.03734 KJ/Kg` (-ve sign shows heat is getting out)

Part B:

From Table A-23:

`u_(1) at 320K = 7526 KJ/Kg`

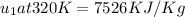
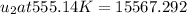`u_(2) at 555.14K = 15567.292` (By interpolation)

`Q=m((u(T_(2))-u(T_(1)))/(M) )+W_(12)`

`Q=0.30338((15567.292-7526)/(44.01) )+(-53.9056)`

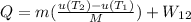
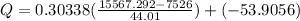
`Q=1.5265KJ/Kg` (Heat getting in)

The value of Q at constant specific heat is approximately 361% in difference with variable specific heat and at constant specific heat Q has opposite direction (going in) than Q which is calculated in Part B from table A-23. So taking constant specific heat is not a good idea and is questionable.