Answer:
250 days.
Explanation:
Let x represent number of days.
We have been given that of the 2,500 tons of ore mined daily at a quarry, 0.4 percent results in a certain pure metal.
Let us find the amount of pure metal by calculating 0.4% of 2500.
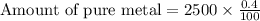


Amount of pure metal mined in x days will be
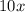 .
.
Since we need total pure metal production equal to 2500 ton, so we can set an equation as:
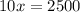
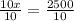
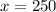
Therefore, it will take 250 days of mining for the total amount of pure metal produced at the quarry to be equal to the daily amount of ore mined.