Question

Find the sum of the measures of the interior angles:

1. Nonagon
2.Pentagon
3.11-gon
4.18.gon
5.38-gon
6.56-gon

Please show all work on how you got each answer

Answer 1

The sum of the measures of the interior angles

The sum of interior angles of any polygon can be foud wuth the following general formula

Sum of interior angles = (n-2) \ times 180^{\circ}

where n is the number of sides

1. Nonagon

The Nonagon is a polygon with 9 sides. then,

n = 9

Sum of interior angles of Nonagon

=
`(9-2) * 180^(\circ)`

=
`(7) * 180^(\circ)`

=
`1260^(\circ)`

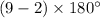
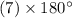
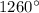
2.Pentagon

The Pentagon is a polygon with 5 sides. then,

n = 5

Sum of interior angles of Pentagon

=
`(5-2) * 180^(\circ)`

=
`(3) * 180^(\circ)`

=
`540^(\circ)`

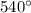
3. 11-gon

The 11-gon is a polygon with 11 sides. then,

n = 11

Sum of interior angles of 11-gon

=
`(11-2) * 180^(\circ)`

=
`(9) * 180^(\circ)`

=
`1620^(\circ)`

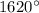
4. 18-gon

The 18-gon is a polygon with 18 sides. then,

n = 18

Sum of interior angles of 18-gon

=
`(18-2) * 180^(\circ)`

=
`(16) * 180^(\circ)`

=
`2880^(\circ)`

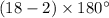
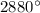
5. 38-gon

The 38-gon is a polygon with 38 sides. then,

n = 38

Sum of interior angles of 38-gon

=
`(38-2) * 180^(\circ)`

=
`(36) * 180^(\circ)`

=
`6480^(\circ)`

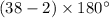
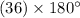
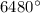
6.56-gon

The 56-gon is a polygon with 56 sides. then,

n = 56

Sum of interior angles 56-gon

=
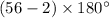
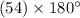
`(56-2) * 180^(\circ)`

=
`(54) * 180^(\circ)`

=
`9720^(\circ)`