Question

A tower that is 166 feet tall casts a shadow 167 feet long. Find the angle of elevation of the sun to the

nearest degree.

Answer 1

The angle is approximately
`45^\circ`.

Explanation:

We should start with a diagram (like the one attached). Notice the length of the tower and the length of the shadow are the legs of a right triangle, and the angle of elevation is an acute angle of that triangle.

Since we are given the opposite and adjacent sides to the angle, we will use the tangent function:

`tan(\theta)=\frac{\text{opposite side}}{\text{adjacent side}}`

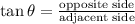
In this case, the opposite side is the height of the tower, 166 ft. The adjacent side is the length of the shadow, 167 ft. So, we have:

`tan(\theta)=(166)/(167)`

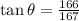
To get the angle, we need to use inverse tangent:

`\theta=\tan^(-1)((166)/(167))\\\theta \approx 45`

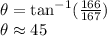
The angle is approximated
`45^\circ` when rounded to the nearest degree.