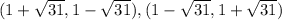Question

Find the points where the line y = − x 2 intersects a circle of radius 8 centered at the origin. Give exact values for the x and y coordinates.

Answer 1

So the coordinates are
`(1+ √(31), 1-√(31)),(1-√(31) , 1+√(31))`

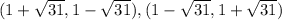
Explanation:

For this case we assume that the line is given by this formula
`y = 2-x`

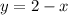
We know that the general equation for a circle is given by:

`(x-h)^2 +(y-k)^2 = r^2`

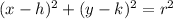
Since the circle for this case is centered at the origin then h,k =0 and we have this:

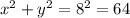`x^2 + y^2 = 8^2 = 64` (1)

For this case we can replace the formula for y from the line into equation (1) and we got:

`x^2 + (2-x)^2= 64`

We know from algebra that
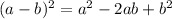`(a-b)^2 = a^2 -2ab +b^2` if we use this concept we got:

`x^2 +4 -4x + x^2 = 64`

We can subtract 64 on both sides and we got:

`2x^2 -4x -60 =0`

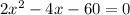
Now we can divide both sides by 2 and we got:

`x^2 -2x -30 = 0`

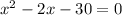
And we can use the quadratic formula to solve this:

`x = (-b \pm √(b^2 -4ac))/(2a)`

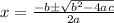
For this case
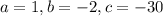`a=1, b=-2 , c=-30` and if we replace we got:

`x = (2 \pm √(2-4*(1)*(-30)))/(2)`

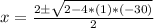
And we got
`x_1= 1+√(31) , x_2 = 1-√(31)`

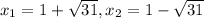
Now we just need to replace into the original equation for the line and we get the y coordinates like this:

`y_1 = 2- (1+√(31)) =1-√(31)`

`y_2 = 2- (1-√(31)) =1+√(31)`

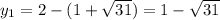
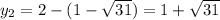
So the coordinates are
`(1+ √(31), 1-√(31)),(1-√(31) , 1+√(31))`