Option A
-x + 6y = 42 is the standard form
Solution:
Given that we have to write
 in standard form
in standard form
The standard form of an equation is Ax + By = C
In this kind of equation, x and y are variables and A, B, and C are integers
Given equation is:

Let us convert the above equation into standard form
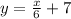
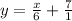
Make the denominator same in R.H.S
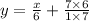
Solve the above equation
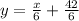
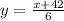
Move 6 from R.H.S to L.H.S

Bring all the terms to one side, leaving only constant on R.H.S
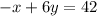
The above equation is of form Ax + By = C
Thus option A is correct