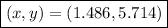Answer:
At point (1.486, 5.714)
Explanation:
The equation of the circle with radius 4 and center at (0, 2) is:
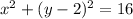
and we want to know where in the 1st quadrant does it intersect with line
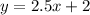
To find the point of intersection we put the value of
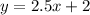 into the equation of the circle:
into the equation of the circle:
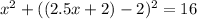
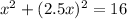

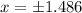
and since we only concerned with the 1st quadrant we take the positive value of
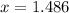 which is the x coordinate of intersection.
which is the x coordinate of intersection.
The y coordinate is found by putting this x value into either the circle equation or the line equation ; we choose the line equation
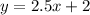 because it is easier to work with.
because it is easier to work with.
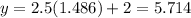
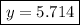
Thus we have the point of intersection