Question

Many newspapers cerry a certain puzzle in which the reader must unscramble letters to form words. How many ways can the letters of RTLIOSN be arranged? Identify the correct unscrambling, then determine the probability of getting the result be randonly slecting one arrangement of the given letters.

How many ways cant eh letters of RTLIOSN be arranged?
What is the correct unscrambling or RTLIOSN?
What is the probability of coming up with the correct unscrambling throughrandom letter selection?

Answer 1

a)
`7! = 7*6*5*4*3*2*1`

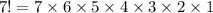
b) NOSTRIL

c)
`P(\text{NOSTRIL}) = (1)/(5040)\approx0.000198`

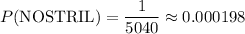
Explanation:

The word is RTLIOSN.

a) this is a 7 letter word, and no letters are repeating.

a short answer is:
`7! = 7*6*5*4*3*2*1`

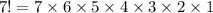
! : is a factorial.

the reason behind this is that we calculated all the possibilities of the 7 lettered word. Let's start by have 7 places where letter could take place.

_ _ _ _ _ _ _

at the first position (doesn't matter which particular position), any letter can take place, right? so here there are 7 possibilities

7 _ _ _ _ _ _

Now since one letter has already been used, the possibilities for the second position now 7 anymore, its the amount of the remaining letters, i.e 6.

7 6 _ _ _ _ _

and we'll continue this until all position are filled.

7 6 5 4 3 2 _

Its clear that when when one position is left its means that all letters have been used except one, so there's only one possible letter that take the last place, hence there's only one possibility

7 6 5 4 3 2 1. Now we multiply all the possibilities and the answer is 7!.

b) NOSTRIL

c) Probability is defined as:

`P(\text{desired result(s)}) = \frac{\text{number of desired result(s)}}{\text{number of all results}}`

here our desired result is to get the correct unscrambling or NOSTRIL. Only this one arrangement of letters result in the correct unscrambling

and all results are all the possible arrangement of the letters RTLIOSN

`P(\text{NOSTRIL}) = \frac{\text{\#arrangements that result in NOSTRIL}}{\text{\#all results}}`

`P(\text{NOSTRIL}) = (1)/(7!)`

`P(\text{NOSTRIL}) = (1)/(5040)\approx0.000198`

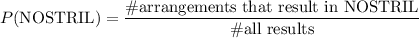
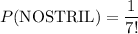
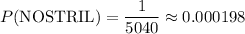
So, by random letter selection, the probability of getting the correct unscrambling of the letters RTLIOSN, i.e. NOSTRIL, is 1 out of 5040 trials or 0.000198 or 0.0198%