Answer:
x = 30
y = 59.33
Explanation:
We have Redrawn the diagram by naming the quadrilateral.
Now According to redrawn diagram;
∠ A = 90°
∠ B =

∠ C =
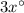
∠ D =
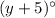
We need to find the value of 'x' and 'y'.
Solution:
Now we know that;
"If a quadrilateral whose all four sides or vertices touches the circle then it is said to be cyclic quadrilateral."
The Given diagram is a Cyclic Quadrilateral.
Now By Properties of Cyclic Quadrilateral which states that;
"Sum of the angles of opposite side of quadrilateral is always 180°."
So we can say that;
∠ A + ∠ C = 180°
∠ B + ∠ D = 180°
Substituting the values we get;

Also
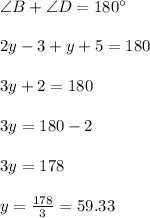
Hence The Value of x is 30 and y is 59.33.