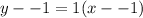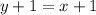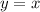Question

Write in point-slope form the equation of the line that is parallel to the given line and passes through the given point.Your final answer should be in slope-intercept form.1. y= x+ 5, (-1, -1)

Answer 1

`y = x`

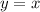
Explanation:

The given equation is

`y = x + 5`

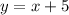
The slope of this line

`m = 1`

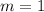
Since all parallel lines have the same slope, the slope of our line is also m=1.

We now use the point-slope formula

`y-y_1=m(x-x_1)`

Since the line passes through (-1,-1)

We gave

`x_1=-1,y_1=-1`

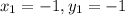
We substitute the slope and point to get

`y - - 1 = 1(x - - 1)`

`y + 1 = x + 1`

`y = x`