Answer: 0.8461
Explanation:
Let p be the proportion of residents are against the increase of taxes to fund alternatives to drug addiction treatment.
As per given , we have p=0.40
A random sample is taken with size : n= 400
Expecting sample proportion :

Now , the probability that more than 150 of the residents surveyed will be against increasing taxes if a random sample of 400 residents are surveyed will be :
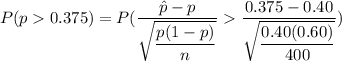
![=P(z>\frac{0.375-0.40}{\sqrt{(0.40(0.60))/(400)}})\ \ [\because\ z=\frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}]](https://img.qammunity.org/2021/formulas/mathematics/college/lj4evurdbvm4m5g0sy5hwqo70m5zmgb78i.png)
![=P(z>-1.02)=P(z<1.02)\ \ [\because \ P(Z>-z)=P(Z<z)]](https://img.qammunity.org/2021/formulas/mathematics/college/245gl5saauqkk1vlxe93h8ly5dfikac7qz.png)
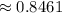 [By z-table]
[By z-table]
Hence, the approximate probability that more than 150 of the residents surveyed will be against increasing taxes if a random sample of 400 residents are surveyed is 0.8461 .