Answer:
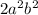
Explanation:
Step 1: The given expression is 2·a·b·a·b.
Dot represents multiplication.
Step 2: To simplify the expression, arrange like terms into one group
2·a·b·a·b = 2·(a·a)·(b·b)
Step 3: Law of exponents:
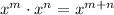
Using the law of exponents formula, we get
2·(a·a)·(b·b) =
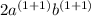
=
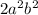
Therefore, 2·(a·a)·(b·b) =
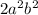
Hence, the simplified form of 2·(a·a)·(b·b) is
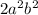 .
.