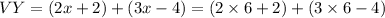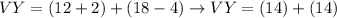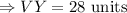The measure of VY is 28 units
Solution:
To find: The measure of VY
From the given,
WZ bisects VY, then we can conclude that VZ = ZY ---> (1)
VZ=2x+2 and ZY=3x-4
Substituting the above values in (1) we get,
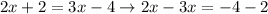
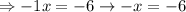
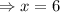
Since, WZ bisects VY, VY=VZ+ZY ---> (2)
On substituting the value of x in (2) we get,