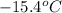Question

A certain substance X has a normal freezing point of -10.1 degree C and a molal freezing point depression constant Kf = 5.32 °C.kg. mol^-1. Calculate the freezing point of a solution made of 29.82 g of urea ((NH2)2CO) dissolved in 500.

Answer 1

Answer : The freezing point of a solution is
`-15.4^oC`

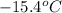
Explanation : Given,

Molal-freezing-point-depression constant
`(K_f)` =
`5.32^oC/m`

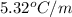
Mass of urea (solute) = 29.82 g

Mass of solvent = 500 g = 0.500 kg

Molar mass of urea = 60.06 g/mole

Formula used :

`\Delta T_f=i* K_f* m\\\\T^o-T_s=i* K_f*\frac{\text{Mass of urea}}{\text{Molar mass of urea}* \text{Mass of solvent in Kg}}`

where,

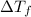`\Delta T_f` = change in freezing point

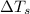`\Delta T_s` = freezing point of solution = ?

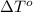`\Delta T^o` = freezing point of solvent =
`-10.1^oC`

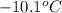
i = Van't Hoff factor = 1 (for urea non-electrolyte)

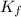`K_f` = freezing point constant =
`5.32^oC/m`

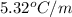
m = molality

Now put all the given values in this formula, we get

`-10.1^oC-T_s=1* (5.32^oC/m)* (29.82g)/(60.06g/mol* 0.500kg)`

`T_s=-15.4^oC`

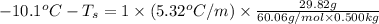
Therefore, the freezing point of a solution is
`-15.4^oC`