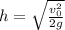Answer:
Maximum height will be
Step-by-step explanation:
We have given initial velocity through which basketball is thrown
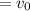
Acceleration due to gravity
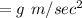
At maximum height velocity will be zero
So final velocity v = 0 m /sec
According to third equation of motion
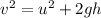
 ( Negative sign is due to upward acceleration )
( Negative sign is due to upward acceleration )