Question

A tennis ball is thrown off a cliff at an angle of 26° above the horizontal. The tennis ball hits the ground below the cliff 3.2 seconds later at a horizontal distance of 200 ft from where it was thrown. How high is the cliff?

Answer 1

h = 56.57 ft

Step-by-step explanation:

given,

angle at which tennis ball was hit = 26°

time taken to hit the cliff = 3.2 s

horizontal distance = 200 ft.

calculation of velocity

horizontal velocity,v_x = v cos θ

distance = speed x time

200 = v cos 26° x 3.2

v = 69.54 ft/s

now, calculation of time to reach maximum height

final velocity = 0 ft/s

vertical velocity = v sin θ

using equation of motion

v = u + at

0 = v sin 29° - g t

32.2 t = 69.54 sin 29°

t = 1.053 s

vertical distance of maximum height travel by the ball

`s = u t +(1)/(2)gt^2`

`x= 69.54* sin 29^0* 1.053-(1)/(2)* 32.2* 1.053^2`

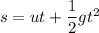
x = 17.64 ft

now,

height of the cliff

distance traveled by the ball from top point to ground= x + h

time taken = t' = 3.2 - 1.053 = 2.147 s

initial velocity = 0 ft/s

using equation of motion

`s = u t +(1)/(2)gt^2`

`x + h =0 +(1)/(2)* 32.2* 2.147^2`

`17.64 + h =74.21`

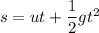
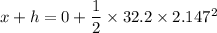
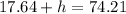
h = 56.57 ft

height of the cliff is equal to 56.57 ft