Question

With no stops, it takes 3 hours for a motorist to make a trip driving at an average speed of 65 mph. When the motorist stops for gas and a sandwich on the same trip, he averages 60 mph. How many minutes long is the stop?

Answer 1

The time for which motorist stop for gas and sandwich is 15 minutes

Explanation:

Given as :

Let The distance cover by motorist = d miles

Let The time for which motorist stop = n minutes

The time taken by motorist to cover d distance with no stop = t = 3 hours

The average speed of motorist with no stop = s = 65 miles per hour

Again

The time taken by motorist to cover d distance with stop = T hours

The average speed of motorist with stop = S = 60 miles per hour

∵ Distance = Speed × Time

So, with no stop

d = s × t

Or, d = 65 miles per hour × 3 hours

Or, d = 195 miles

And, with stop

d = S × T

Or, d = 60 miles per hour × T hours

∵ d = 195 miles

So, 60 miles per hour × T hours = 195 miles

Or , 60 T = 195

∴ T =
`(195)/(60)`

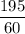
i.e T = 3.25 hours

Or, T = 3 hours +
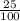`(25)/(100)` hours

Or, T = 3 hours +
`(1)/(4)` hours

Or, T = 3 hours +
`(60)/(4)` minutes

Or, T = 3 hours + 15 minutes

So, time taken by motorist to cover d distance with stop = T = 3 h + 15 min

Now,

The time for which motorist stop = time taken by motorist to cover d distance with stop - time taken by motorist to cover d distance with no stop

i.e n = T - t

Or, n = ( 3 hours + 15 minutes) - ( 3 hours)

∴ n = 15 minutes

So, The time for which motorist stop = n = 15 minutes

Hence, The time for which motorist stop for gas and sandwich is 15 minutes . Answer