Question

the value of k so that the line Containing the points (-8,k) and (-4,-8) is perpendicular) to the line containing the points (10, -11) and (5,-5)

Answer 1

`k= (-34)/(3)`

Explanation:

Step 1 :-

Perpendicular condition :-

Step 1:-

Two non-vertical lines are perpendicular to each other if and only if their slopes are negative reciprocals of each other.

`m_(2) = (-1)/(m_(1) )`

`m_(1) m_(2) = -1`

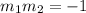
Step 2:-

The given points are (-8,k) and (-4,-8)

`m_(1) = (y_(2)-y_(1)  )/(x_(2)-x_(1)  )`

finding slope of the first line is
`m_(1)`

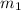
using formula
`m_(1) = (-8-k)/(-4+8)`

=
`(-8-k)/(4)`

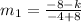
finding slope of the second line is
`m_(2)`

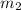
using formula
`m_(2) = (--5+11)/(5-10)`

=
`(6)/(-5)`

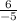
Step 3:-

using perpendicular condition

The two lines are perpendicular and their slopes are

`m_(1) m_(2) = -1`

`((-k-8)/(4) )((-6)/(5) )= -1`

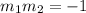
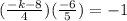
simplification,we get solution is

`(6(k+8))/(20) =-1`

`6 k+48 =-20`

`6 k = -20 -48`

`6 k = -68`

`(-68)/(6)`

`k= (-34)/(3)`

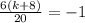
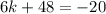
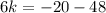
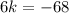
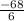
Final answer is

`k= (-34)/(3)`