Answer:
Option B) Yes, because its corresponding z score is greater than 2.
Explanation:
We are given the following information in the question:
Mean, μ = 268.3 days
Standard Deviation, σ = 15.1 days
We are given that the distribution of lengths of pregnancies is a bell shaped distribution that is a normal distribution.
Formula:

For x = 310
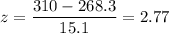
Since the obtained z score is greater than 2 we could that 310 is unusual.
Option B) Yes, because its corresponding z score is greater than 2.