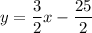Question

Equation in point slope form that passes through (3,-8) and 7(-2)

Answer 1

Therefore the equation of the line through ( 3, -8 ) and ( 7, -2) is

`y=(3)/(2)x-(25)/(2)`

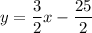
Explanation:

Given

point A( x₁ , y₁) ≡ ( 3 ,-8)

point B( x₂ , y₂) ≡ (7 , -2)

To Find:

Equation of Line AB =?

Solution:

Equation of a line passing through Two points A( x₁ , y₁) and B( x₂ , y₂)is given by the formula

`(y - y_(1) )=((y_(2)-y_(1) )/(x_(2)-x_(1) ))*(x-x_(1)) \\`

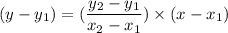
Substituting the given values in a above equation we get

`y-(-8)=(-2-(-8))/(7-3)* (x-3)\\\\y+8=(3)/(2)(x-3)\\\\2(y+8)=3(x-3)\\\\2y+16=3x-9\\y=(3)/(2)x-(25)/(2)`

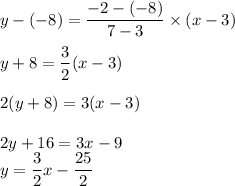
Which is in Point-Slope Form i,e

`y =mx +c`

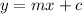
Where m = slope , and c = y - intercept

Therefore the equation of the line through ( 3, -8 ) and ( 7, -2) is

`y=(3)/(2)x-(25)/(2)`