Question

The US Department of Energy reported that 48% of homes were heated by natural gas. A random sample of 333 homes in Oregon found that 149 were heated by natural gas. Test the claim that proportion of homes in Oregon that were heated by natural gas is different from what was reported. Use a 1% significance level.

a) What are the correct hypotheses?

A. H0 : μ=0.48 H1 : μ≠0.48

B. H0 : p=0.45 H1 : p≠0.45

C. H0 : p≠0.48 H1 : p=0.48

D. H0 : p=0.48 H1 : p≠0.48

E. H0 : p=0.48 H1 : p>0.48

b) The p-value is 0.2344. Choose the correct decision and summary.

A. Reject H0, there is not enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

B. Do not reject H0, there is not enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

C. Reject H0, there is enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

D. Do not reject H0, there is enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

Answer 1

The correct hypotheses for the statistical test are H0: p = 0.48 and H1: p ≠ 0.48. With a p-value of 0.2344 and a 1% significance level, we do not reject the null hypothesis, indicating insufficient evidence to support the claim that the proportion of homes in Oregon using natural gas for heating differs from the reported 48%.

Step-by-step explanation:

The correct hypotheses for testing the claim that the proportion of homes in Oregon heated by natural gas is different from what was reported by the US Department of Energy are:

H0: p = 0.48and

H1: p ≠ 0.48

.This corresponds to option D. The null hypothesis (H0) states that there is no difference between the Oregon proportion and the reported proportion, while the alternative hypothesis (H1) states that there is a difference.

The decision based on the p-value of 0.2344 at a 1% significance level is:

B. Do not reject H0

,since the p-value is higher than the significance level of 0.01. Hence, there is not enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

Answer 2

Option B) Do not reject null hypothesis, there is not enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.

Step-by-step explanation:

We are given the following in the question:

Sample size, n = 333

p = 48% = 0.48

Alpha, α = 0.01

Number of women belonging to union , x = 149

First, we design the null and the alternate hypothesis

`H_(0): p = 0.48\\H_A: p \\eq 0.48`

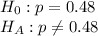
This is a two-tailed test.

Formula:

`\hat{p} = (x)/(n) = (149)/(333) = 0.45`

`z = \frac{\hat{p}-p}{\sqrt{(p(1-p))/(n)}}`

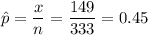
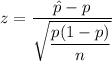
Putting the values, we get,

`z = \displaystyle\frac{0.45-0.48}{\sqrt{(0.48(1-0.48))/(333)}} = -1.096`

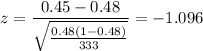
Now, we calculate the p-value from the table.

P-value = 0.273079

Since the p-value is greater than the significance level, we fail to reject the null hypothesis and accept the null hypothesis.

Thus, there is not enough evidence to support the claim that proportion of homes in Oregon that were heated by natural gas is different from what was reported.

B. Do not reject null hypothesis, there is not enough evidence to support the claim that the proportion of homes in Oregon heated by natural gas is different from the 48% that the US Department of Energy reported.