Question

It has been observed that some persons who suffer colitis, again suffer colitis within one year of the first episode. This is due, in part, to damage from the first episode. The performance of a new drug designed to prevent a second episode is to be tested for its effectiveness in preventing a second episode. In order to do this two groups of people suffering a first episode are selected. There are 118 people in the first group and this group will be administered the new drug. There are 127 people in the second group and this group wil be administered a placebo. After one year, 12% of the first group has a second episode and 13% of the second group has a second episode. Select a 90% confidence interval for the difference in true proportion of the two groups. a) [-0.060, -0.043] b) [-0.080, 0.060] c) [-0.093, 0.073] d) [-0.580, 0.560] e) [-0.073, 0.093] f) None of the above

Answer 1

Answer: b) [-0.080, 0.060]

Explanation:

The confidence interval for the difference in true proportion of the two groups. is given by :-

`\hat{p}_1-\hat{p}_2\pm z^* \sqrt{\frac{\hat{p}_1(1-\hat{p}_1)}{n_1}+\frac{\hat{p}_2(1-\hat{p}_2)}{n_2}}`

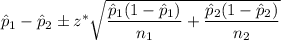
, where
`n_1` = Sample size for first group.

`n_2` = Sample size for second group.

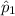`\hat{p}_1` = Sample proportion for first group.

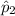`\hat{p}_2` = Sample proportion for second group.

z* = critical z-value.

As per given , we have

`n_1=118` ,
`n_2=127` ,
`\hat{p}_1=0.12` ,
`\hat{p}_2=0.13`

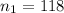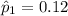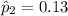
Critical value for 90% confidence interval is 1.645. (By z-table)

Substitute all values in formula , we get

`0.12-0.13\pm (1.645) \sqrt{(0.12(1-0.12))/(118)+(0.13(1-0.13))/(127)}`

`=-0.01\pm (1.645) √(0.000895+0.000890)`

`=-0.01\pm (1.645) √(0.001785)`

`=-0.01\pm (1.645) (0.04225)`

`\approx-0.01\pm 0.070`

`=(-0.01-0.070,\ -0.01+0.070)=(-0.080,\ 0.060)`

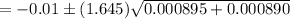
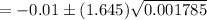
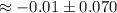
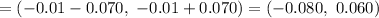
Hence, the 90% confidence interval for the difference in true proportion of the two groups. is (-0.080, 0.060)

Hence, the correct answer is b) [-0.080, 0.060]