Question

A circle with radius 3 has a sector with a central angle of 9/17 π radians .

What is the area of the sector?

Answer 1

The area of sector is 7.48 square units

Solution:

Given that circle has radius 3

Central angle =
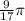`(9)/(17) \pi` radians

To find: area of sector

The area of a sector of a circle is:

`\text{Area of sector } = (1)/(2) r^2 \theta`

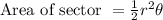
Where, "r" is the radius of circle and
`\theta` is the angle in radians

Substituting the values in formula,

`\text{Area of sector } = (1)/(2) * 3^2 * (9)/(17 ) * \pi\\\\\text{Area of sector } = (1)/(2) * 9 * (9)/(17) * 3.14\\\\\text{Area of sector } = 7.48`

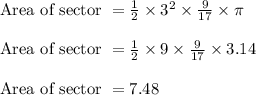
Thus the area of sector is 7.48 square units