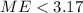Answer:
What would happen to the margin of error if Nina decreases the confidence level to 90%?
On this case since we have the same deviation and sample size the only factor that affect the margin of error is the value of
 and for a 90% the critical value would be lower than for 95% of confidence.
and for a 90% the critical value would be lower than for 95% of confidence.
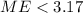
What would happen to the margin of error if Nina increases the confidence level to 99%?
On this case since we have the same deviation and sample size the only factor that affect the margin of error is the value of
 and for a 99% the critical value would be greater than for 95% of confidence.
and for a 99% the critical value would be greater than for 95% of confidence.
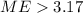
Nina decreases the sample size to 33 locations?
If we see the sample size is on the denominator in the formula for the margin of error, our original sample size was 45, if we decrease to n=33 then the margin of error would be greater than the original.
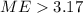
Nina increases the sample size to 65 locations?
If we see the sample size is on the denominator in the formula for the margin of error, our original sample size was 45, if we increase to n=65 then the margin of error would be lower than the original.
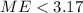
Explanation:
Previous concepts
A confidence interval is "a range of values that’s likely to include a population value with a certain degree of confidence. It is often expressed a % whereby a population means lies between an upper and lower interval".
The margin of error is the range of values below and above the sample statistic in a confidence interval.
Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".
Solution to the problem
The confidence interval for the mean is given by the following formula:
 (1)
(1)
For this case the sample size is n =45
The margin of error is given by this formula:
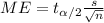 (2)
(2)
And for this case at 95% the margin of error calculated by Nina was
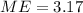
What would happen to the margin of error if Nina decreases the confidence level to 90%?
On this case since we have the same deviation and sample size the only factor that affect the margin of error is the value of
 and for a 90% the critical value would be lower than for 95% of confidence.
and for a 90% the critical value would be lower than for 95% of confidence.
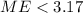
What would happen to the margin of error if Nina increases the confidence level to 99%?
On this case since we have the same deviation and sample size the only factor that affect the margin of error is the value of
 and for a 99% the critical value would be greater than for 95% of confidence.
and for a 99% the critical value would be greater than for 95% of confidence.
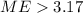
Nina decreases the sample size to 33 locations?
If we see the sample size is on the denominator in the formula for the margin of error, our original sample size was 45, if we decrease to n=33 then the margin of error would be greater than the original.
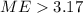
Nina increases the sample size to 65 locations?
If we see the sample size is on the denominator in the formula for the margin of error, our original sample size was 45, if we increase to n=65 then the margin of error would be lower than the original.