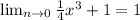Answer:
B. The Limit is 1
Explanation:
Use the sandwhich theorem. The limit at both end as x approaches 0 is equal to 1, therefore f(x) must be equal to 1 as well because it's sandwhich between the two intervals.
Solving the Limits of the Endpoint:
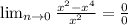
This is an in-determinant form so you must use L'Hopitals rule.