Question

Cloud nine is having a sale on all shirts and pants in the store.Olivia purchased 8 shirts 4 pairs of pants for $176.Raheem purchased 3 shirts and 4 pairs of pants for 116.Let x represent the price of a shirt and y represent the price of a pair of pants. Lwrite a system of equations

Answer 1

Question is Incomplete; Complete question is given below;

Cloud Nine is having a sale on all shirts and pants in the store. Olivia purchased 8 shirts and 4 pairs of pants for $176. Raheem purchased 3 shirts and 4 pairs of pants for $116. Let x represent the price of a shirt and y represent the price of a pair of pants. Write a system of equations, and use the elimination method to find a reasonable price for a pair of pants.

Answer:

The system of equations are
`\left \{ {{8x+4y=176} \atop {3x+4y=116}} \right.`

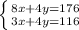
The reasonable price for pair of pants is $20.

Explanation:

Let the price of shirt be 'x'.

Let price of pair of pants be 'y'.

Given:

Olivia purchased 8 shirts 4 pairs of pants for $176

So we can say that;

8 multiplied by the price of shirt plus 4 multiplied by the price of pair of pants is equal to 176.

framing in equation form we get;

`8x+4y=176 \ \ \ \ equation \ 1`

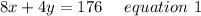
Also Given:

Raheem purchased 3 shirts and 4 pairs of pants for 116.

So we can say that;

3 multiplied by the price of shirt plus 4 multiplied by the price of pair of pants is equal to 116.

framing in equation form we get;

`3x+4y=116 \ \ \ \ equation \ 2`

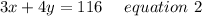
Hence the system of equation are
`\left \{ {{8x+4y=176} \atop {3x+4y=116}} \right.`

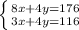
Now by using elimination method we will subtract equation 2 from equation 1 we get;

`(8x+4y)-(3x+4y)=176-116\\\\8x+4y-3x-4y = 60\\\\5x =60\\\\x=(60)/(5)=\$12`

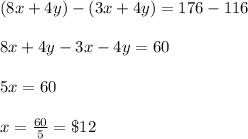
Now Substituting the value of x in equation 1 we get;

`8x+4y=176\\\\8* 12+4y =176\\\\96+4y=176\\\\4y =176-96\\\\4y = 80\\\\y= (80)/(4) =\$ 20`

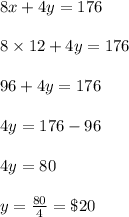
Hence The reasonable price for pair of pants is $20.