Question

At westwood Middleschool, 648 students responded to a survey asking about their favorite type of exercise. Twice as many students chose jogging as swimming. The same number of students chose yoga as jogging. Twice as many students chose biking as jogging. How many students chose each type of exercise?

Answer 1

The number of students chose jogging is 144, chose swimming is 72, chose yoga is 144 and chose biking is 288.

Explanation:

Given:

At westwood Middle School, 648 students responded to a survey asking about their favorite type of exercise.

Twice as many students chose jogging as swimming.

The same number of students chose yoga as jogging.

Twice as many students chose biking as jogging.

Now, to find the number of students chose each type of exercise.

Let the students chose jogging be
`w.`

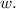
The student chose swimming be
`x.`

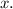
The student chose yoga be
`y.`

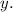
And the student chose biking be
`z.`

As per given in question:

Twice as many students chose jogging as swimming.

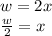`w=2x\\(w)/(2) =x\\`.........(1)

The same number of students chose yoga as jogging.

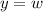`y=w` .........(2)

Twice as many students chose biking as jogging.

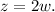`z=2w.` .........(3)

Total students surveyed = 648.

According to question:

`w+x+y+z=648.`

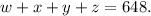
Putting the value from above equations (1), (2) and (3) we :

`w+(w)/(2) +w+2w=648`

`4w+(w)/(2)=648`

`(8w+w)/(2) =648`

`(9w)/(2)=648`

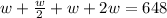
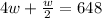
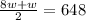
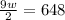
Multiplying both sides by 2 we get:

`9w=1296`

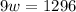
Dividing both sides by 9 we get:

`w=144.`

The students chose jogging = 144.

The student chose swimming:

`x=(w)/(2) \\x=(144)/(2) \\x=72`

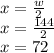
The students chose yoga:

`y=w\\y=144.`

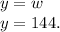
The student chose biking :

`z=2w\\z=2* 144\\z=288`

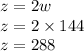
Therefore, the number of students chose jogging is 144, chose swimming is 72, chose yoga is 144 and chose biking is 288.