Question

Analyzing a Graph In Exercise, analyze and sketch the graph of the function. Lable any relative extrema, points of inflection, and asymptotes. See Example 6.

y = ln 5x/x^2

Answer 1

Analyzed and Sketched.

Explanation:

We are given
`y=(\ln\left(5x))/(x^2)`

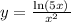
To sketch the graph we need to find 2 components.

1) First derivative of y with respect to x to determine the interval where function increases and decreases.

2) Second derivative of y with respect to x to determine the interval where function is concave up and concave down.

`y'=(1-2\ln\left(5x))/(x^3)=0`

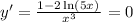
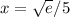`x = \sqrt e/5` is absolute maximum

`y''=(6\ln\left(5x)-5)/(x^4)=0`

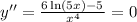
`x=e^(5/6)/5` is the point concavity changes from down to up.

Here, x = 0 is vertical asymptote and y = 0 is horizontal asymptote.

The graph is given in the attachment.