Question

Solving Exponential and Logarithmic Equation In exercise,solve for x or t.See example 5 and 6.

4e2x - 1 -1 = 5

Answer 1

0.70275

Explanation:

Data provided in the question:

4e²ˣ⁻¹ -1 = 5

now solving for 'x'

⇒ 4e²ˣ⁻¹ = 5 + 1

or

⇒ 4e²ˣ⁻¹ = 6

or

⇒
`e^((2x-1)) =(6)/(4)`

or

⇒
`e^((2x-1)) =(3)/(2)`

now,

taking natural log both sides, we get

⇒ 2x - 1 =
`\ln((3)/(2))`

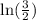
also,

we know

⇒
`\log((A)/(B)) = \log(A)+\log(B)`

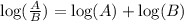
thus,

⇒ 2x - 1 = ln(3) - ln(2)

or

⇒ 2x - 1 = 1.0986 - 0.6931

or

⇒ 2x - 1 = 0.4055

or

⇒ 2x = 0.4055 + 1

or

⇒ 2x = 1.4055

or

⇒ x = 0.70275