We can use three rules to solve this logarithm:
Power rule:
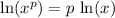
Product rule:
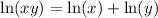
Quotient rule:
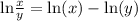
Simplify each part of the logarithm:
4 In x → ln(x^4)
6 In y → ln(y^6)
In z → ln(z)
We multiply ln(x^4) and ln(y^6) according to the product rule and we divide it by ln(z) according to the quotient rule.
Therefore, the logarithm as a single quantity is
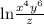
Best of Luck!