Question

For what value(s) of k is the linear system consistent? (Enter your answers as a comma-separated list.)

6x1 − 9x2 = 8

9x1 + kx2 = −1

x1 & x2 are subscripts

Answer 1

`k\\eq -(27)/(2)`

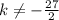
Explanation:

We have been given a system of equations as
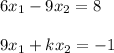`6x_1-9x_2 = 8\\\\9x_1+kx_2 =-1`. We are asked to find the value of k such that the given system is consistent.

Let us consider two equations as:

`a_1x+b_1y = c_1\\\\a_2x+b_2y =c_2`

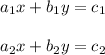
For a system to be consistent the ratio of coefficient of x terms and y terms should not be equal that is:

`(a_1)/(a_2)\\eq (b_1)/(b_2)`

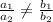
Upon substituting our given values, we will get:

`(6)/(9)\\eq (-9)/(k)`

`6k\\eq -81`

`(6k)/(6)\\eq -(81)/(6)`

`k\\eq -(27)/(2)`

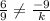
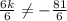
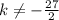
Since the given system will be consistent for all value except
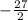`-(27)/(2)`, therefore, we can choose any values for k such as
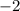`-2` or 2.

Answer 2

Explanation:

Given

`6x_1-9x_2=8`

`9x_1+kx_2=-1`

The given system is
`AX=B` can be represented by

`\begin{bmatrix}6 &-9 \\ 9 & k\end{bmatrix}\begin{bmatrix}x_1\\ x_2\end{bmatrix}=\begin{bmatrix}8\\ -1\end{bmatrix}`

The given system is consistent when determinant of A is not equal to zero

`|A|`

`|A|=6k-(-81)=6k+81`

`k\\eq (-27)/(2)`

i.e. system is consistent for all value of k except
`k=(-27)/(2)`

`R-(-27)/(2)`