Question

Parallelogram P was dilated to form parallelogram P.

What is the value of x? (Figures not drawn to scale.)

Answer 1

X=1

Explanation:

Half of 20 is 10 so half of 8 is 4. Therefore, X(1)+3=4

Answer 2

`x=1`

Explanation:

Given:

Parallelogram P is dilated to form parallelogram P'.

The side of P which is = 10 units corresponds 20 units in P'.

The side of P which is given as =(x+3) units corresponds 8 units in P'.

To find the value of
`x`.

Solution:

The scalar factor of dilation from P to P' can be given as the ratio of the corresponding sides of the parallelograms.

The scalar factor :

⇒
`\frac{\textrm{Side on parallelogram P'}}{\textrm{Side on parallelogram P}}`

⇒
`(20)/(10)`

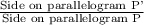
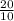
⇒ 2

This means the sides of the parallelogram P' is twice the sides of the parallelogram P.

The side of P which is given as =(x+3) units corresponds 8 units in P'.

Using the scalar factor the equation to solve for
`x` can be given as:

⇒
`2(x+3)=8`

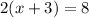
Solving for
`x`.

Using distribution:

⇒
`2x+6=8`

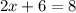
Subtracting both sides by 6.

⇒
`2x+6-6=8-6`

⇒
`2x=2`

Dividing both sides by 2.

⇒
`(2x)/(2)=(2)/(2)`

∴
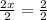
`x=1` (Answer)