Question

As we increase the quantum number of an electron in a one-dimensional, infinite potential well, what happens to the number of maximum points in the probability density function?

Answer 1

we can see that the maximum number increases linearly with the atomic number

Step-by-step explanation:

The wave function of a well of infinite potential is

φ (x) = A sin (n π x / L)

Donate A is the amplitude, n the quantum number and L the width of the well

The energy is given by

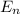`E_(n)` = (h² / 8m L²) n²

With n = 1, 2, 3, …

The probability density is

P = φ*φ

P = A² sin² (n π x / L)

To have maximum intensity the function must be ±-1, so the argument must have ±π /2 rad

n π x / L = π / 2

n = L / 2x

Where x varies between zero and L

For case n = 1, it has only one maximum

For n = 2 it has two maximums,

For n = 3 it has three maximums,

For n = 4 it has 4 maximums

we can see that the maximum number increases linearly with the atomic number