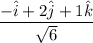Answer: The required unit vector would be
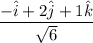
Explanation:
Since we have given that
Let a be the vector which has the same direction as
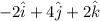 and has length 6.
and has length 6.
Since they are in the same direction so, both have same length i.e. magnitude.
So, the unit vector of a would be
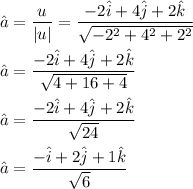
Hence, the required unit vector would be