Answer:
(a) 81 items
(b) 104 items
(c) 155 items
Explanation:
Given:
The expression for the number of items produced by a new employee daily after 't' days on the job is given as:
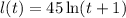
(a) Number of days (t) = 5
Average number of items produced can be obtained by plugging in 5 for 't' in the above expression. This gives,
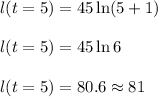
Therefore, nearly 81 items are produced after 5 days.
(b) Number of days (t) = 9
Average number of items produced can be obtained by plugging in 9 for 't' in the above expression. This gives,
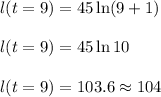
Therefore, nearly 104 items are produced after 9 days.
(c) Number of days (t) = 30
Average number of items produced can be obtained by plugging in 30 for 't' in the above expression. This gives,
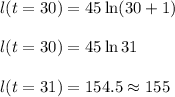
Therefore, nearly 155 items are produced after 30 days.