Answer:
The horizontal asymptote for the function f(x) is 1/2
Explanation:
We were given the function
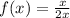
which could be simplified as

this means that the function is a constant of value 1/2 for all value of x.
Then, in particular, the value of f will be 1/2 for x when x tends to infinite and minus infinite.
And because to find the horizontal asymptote of a function we have to calculate the limit of the function when x tends to infinite or minus infinite, we can ensure that this asymptote has also the value 1/2 of f.