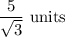Question

Show that the equation represent a sphere, and find its center and radius. 3x2+3y2+3z2=10+6y+12z

Answer 1

Center of sphere = (0, 1, 2)

Radius of sphere =
`(5)/(\sqrt3)\text{ units}`

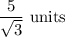
Explanation:

We are given the following in the question:

Equation of sphere:

`3x^2+3y^2+3z^2=10+6y+12z`

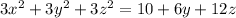
Formula:

The equation of sphere is of the form

`(x-a)^2 + (y-b)^2 + (z-c)^2 = r^2\\\text{where (a,b,c) is the centre of sphere and r is the radius of sphere.}`

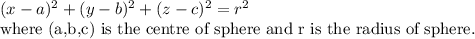
Simplifying the given equation we get,

`3x^2+3y^2+3z^2=10+6y+12z\\\text{Dividing by 3}\\x^2 + y^2 + z^2 = (10)/(3) + 2y + 4z\\\\x^2 + y^2 -2y + z^2-4z = (10)/(3)\\\\\text{Adding 1 and 4 on both sides, we get,}\\\\x^2 + y^2 -2y +1 + z^2-4z + 4 = (10)/(3) + 1+ 4\\\\(x-0)^2 + (y-1)^2 + (z-2)^2 = (25)/(3)\\\\\text{Comparing with the equation of sphere}\\a = 0\\b = 1\\c = 2\\\\r^2 = (25)/(3)\\\\r = \sqrt{(25)/(3)}= (5)/(\sqrt3)`

Center of sphere = (0, 1, 2)

Radius of sphere =
`(5)/(\sqrt3)\text{ units}`