(-4, 1) is the solution to given inequality
Solution:
Given inequality is:
y > | x | - 5
The modulus of a real number x, denoted |x|, is the non-negative value of x without regard to its sign
Let us check the options
Option A
Substitute (x, y) = (4, -1) in given inequality and check if it satisfies the inequality
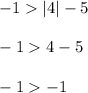
-1 is equal to -1
Thus the inequality is not satisfied
Thus (4, -1) is not the solution
Option B
Substitute (x, y) = (-1, -4) in given inequality
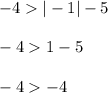
But -4 is equal to -4
So the inequality is not satisfied. Thus (-1, -4) is not a solution
Option C
Substitute (x, y) = (-4, 1) in given inequality

1 is greater than -1
Thus the inequality is satisfied
Thus (-4, 1) is the solution