Question

Modeling Radioactive Decay In Exercise, complete the table for each radioactive isotope.

Amount Amount
after after
Half-life Initial 1000 10,000
Isotope (In years) quantity years years
14C 5715 5 grams

Answer 1

The amount after 1000 years will be, 4.43 grams.

The amount after 10000 years will be, 1.49 grams.

Step-by-step explanation :

Half-life = 5715 years

First we have to calculate the rate constant, we use the formula :

`k=(0.693)/(t_(1/2))`

`k=\frac{0.693}{5715\text{ years}}`

`k=1.21* 10^(-4)\text{ years}^(-1)`

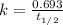
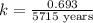
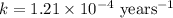
Now we have to calculate the amount after 1000 years.

Expression for rate law for first order kinetics is given by:

`t=(2.303)/(k)\log(a)/(a-x)`

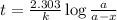
where,

k = rate constant =
`1.21* 10^(-4)\text{ years}^(-1)`

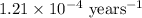
t = time passed by the sample = 1000 years

a = initial amount of the reactant = 5 g

a - x = amount left after decay process = ?

Now put all the given values in above equation, we get

`1000=(2.303)/(1.21* 10^(-4))\log(5)/(a-x)`

`a-x=4.43g`

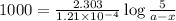
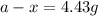
Thus, the amount after 1000 years will be, 4.43 grams.

Now we have to calculate the amount after 10000 years.

Expression for rate law for first order kinetics is given by:

`t=(2.303)/(k)\log(a)/(a-x)`

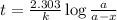
where,

k = rate constant =
`1.21* 10^(-4)\text{ years}^(-1)`

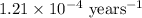
t = time passed by the sample = 10000 years

a = initial amount of the reactant = 5 g

a - x = amount left after decay process = ?

Now put all the given values in above equation, we get

`10000=(2.303)/(1.21* 10^(-4))\log(5)/(a-x)`

`a-x=1.49g`

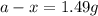
Thus, the amount after 10000 years will be, 1.49 grams.