Answer:
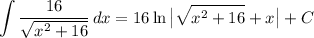
General Formulas and Concepts:
Pre-Calculus
Calculus
Differentiation
- Derivatives
- Derivative Notation
Derivative Property [Multiplied Constant]:
![\displaystyle (d)/(dx) [cf(x)] = c \cdot f'(x)](https://img.qammunity.org/2021/formulas/mathematics/college/bz16ipe6p14y3f6abzxt2zy0j41tg530u9.png)
Integration
- Integrals
- [Indefinite Integrals] integration Constant C
Integration Property [Multiplied Constant]:
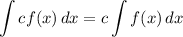
U-Substitution
- Trigonometric Substitution
Explanation:
Step 1: Define
Identify
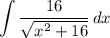
Step 2: Integrate Pt. 1
- [Integral] Rewrite [Integration Property - Multiplied Constant]:
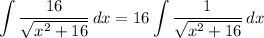
- [Integrand] Rewrite:
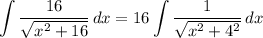
Step 3: Integrate Pt. 2
Set variables for trigonometric substitution.
- Set trigonometric x:
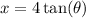
- [x] Differentiate [Trigonometric Differentiation, Multiplied Constant]:
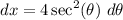
Step 4: Integrate Pt. 3
- [Integral] Trigonometric Substitution:
![\displaystyle \int {(16)/(√(x^2 + 16))} \, dx = 16 \int {(4 \sec ^2(\theta))/(√([4 \tan (\theta)]^2 + 4^2))} \, d\theta](https://img.qammunity.org/2021/formulas/mathematics/college/xyy8ktl90av0bhmyw2fxxjze2g5j0wltw3.png)
- [Integrand] Expand:
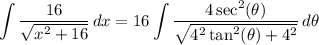
- [Integrand] Factor:
![\displaystyle \int {(16)/(√(x^2 + 16))} \, dx = 16 \int {(4 \sec ^2(\theta))/(√(4^2[ \tan ^2(\theta) + 1]))} \, d\theta](https://img.qammunity.org/2021/formulas/mathematics/college/sh03t6vqzh3oqlc8axga07namjvbrolscz.png)
- [Integrand] Rewrite [Trigonometric Identity]:
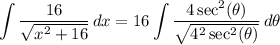
- [Integrand] Simplify:
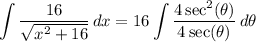
- [Integrand] Simplify:
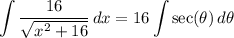
- [Integral] Trigonometric Integration:
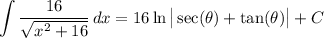
- [Trig] Substitute [See Attachment]:
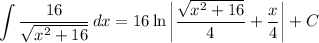
- Simplify:
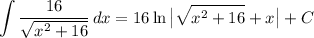
Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Integration