Question

A city park is rectangular in shape. The longer side of the park is 500 feet. A walkway runs

diagonally through the park. The angle formed by the walkway and the shorter side of the park is
65
What is the perimeter of the park?

Answer 1

The perimeter of park is 1466 feet

Solution:

Given that, city park is rectangular in shape

The longer side of park = 500 feet = length of rectangle

Let the shorter side of park be "x" = width of rectangle

The angle formed by the walkway and the shorter side of the park is 65 degrees

Let us first find the width of rectangle

The diagram is attached below

ABCD is rectangle where BC represents the length and DC represents the width

BC = 500 feet

DC = x

Angle D = 65 degrees

Triangle BCD forms a right angled triangle

So by definition of tan, we get

`tan \theta = (opposite)/(adjacent)\\\\tan \theta = (BC)/(DC)\\\\tan 65 = (500)/(x)\\\\x = (500)/(2.14)\\\\x = 233`

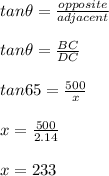
Thus width of rectangle is 233 feet

The perimeter of rectangle is given as:

perimeter = 2(length + width)

perimeter = 2(500 + 233) = 2(733) = 1466

Thus perimeter of park is 1466 feet