Question

Use integration by parts to find the integrals in Exercise.
∫x ln x dx.

Answer 1

`I= (x^2)/(2)lnx- (x^2)/(4)+c`

Explanation:

We have given expression
`\int xln xdx`

Now integrating by part

`I=lnx\int x-\int (d)/(dx)lnx\int x`

`I=lnx* (x^2)/(2)-\int (1)/(x)* (x^2)/(2)`

`I=lnx* (x^2)/(2)-\int (x)/(2)`

`I= (x^2)/(2)lnx- (x^2)/(4)+c`

So integral of the expression will be
`(x^2)/(2)lnx- (x^2)/(4)+c`

Answer 2

`\displaystyle \int {x \ln x} \, dx = (x^2)/(2) \bigg( \ln(x) - (1)/(2) \bigg) + C`

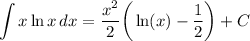
General Formulas and Concepts:

Calculus

Differentiation

• Derivatives
• Derivative Notation

Integration

• Integrals
• [Indefinite Integrals] Integration Constant C

Integration Rule [Reverse Power Rule]:
`\displaystyle \int {x^n} \, dx = (x^(n + 1))/(n + 1) + C`

Integration Property [Multiplied Constant]:
`\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx`

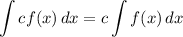
Integration by Parts:
`\displaystyle \int {u} \, dv = uv - \int {v} \, du`

• [IBP] LIPET: Logs, inverses, Polynomials, Exponentials, Trig

Explanation:

Step 1: Define

Identify

`\displaystyle \int {x \ln x} \, dx`

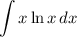
Step 2: Integrate Pt. 1

Identify variables for integration by parts using LIPET.

1. Set u:
   `\displaystyle u = \ln x`
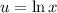
2. [u] Logarithmic Differentiation:
   `\displaystyle du = (1)/(x) \ dx`
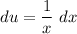
3. Set dv:
   `\displaystyle dv = x \ dx`
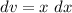
4. [dv] Integration Rule [Reverse Power Rule]:
   `\displaystyle v = (x^2)/(2)`

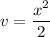
Step 3: Integrate Pt. 2

1. [Integral] Integration by Parts:
   `\displaystyle \int {x \ln x} \, dx = (x^2 \ln x)/(2) - \int {(x)/(2)} \, dx`
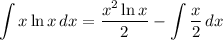
2. [Integral] Rewrite [Integration Property - Multiplied Constant]:
   `\displaystyle \int {x \ln x} \, dx = (x^2 \ln x)/(2) - (1)/(2) \int {x} \, dx`
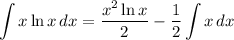
3. [Integral] Integration Rule [Reverse Power Rule]:
   `\displaystyle \int {x \ln x} \, dx = (x^2 \ln x)/(2) - (x^2)/(4) + C`
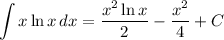
4. Factor:
   `\displaystyle \int {x \ln x} \, dx = (x^2)/(2) \bigg( \ln(x) - (1)/(2) \bigg) + C`

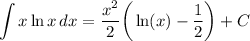
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Integration