Question

At the movies, we had 286 people buy tickets. The adult tickets were $12 and the children's tickets were $4. The total sold was $1968. How many adults and kids attended?

(Please explain the answer algebraically)

Answer 1

103 adults and 183 children's attended

Solution:

Let "a" be the number of adults attended

Let "c" be the number of children attended

Cost of 1 adult ticket = $ 12

Cost of 1 children ticket = $ 4

At the movies, we had 286 people buy tickets

Therefore,

number of adults attended + number of children attended = 286

a + c = 286 ---- eqn 1

The total sold was $1968

Therefore,

number of adults attended x Cost of 1 adult ticket + number of children attended x Cost of 1 children ticket = 1968

`a * 12 + c * 4 = 1968`

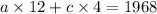
12a + 4c = 1968 ------- eqn 2

Let us solve eqn 1 and eqn 2

From eqn 1,

a = 286 - c -- eqn 3

Substitute eqn 3 in eqn 2

12(286 - c) + 4c = 1968

3432 -12c + 4c = 1968

-8c = 1968 - 3432

-8c = -1464

8c = 1464

c = 183

Substitute c = 183 in eqn 3

a = 286 - 183

a = 103

Thus 103 adults and 183 children's attended