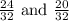The pair of fractions as a pair of fractions with a common denominator of 3/4 and 5/8 is
Solution:
We have to write the given pair of fractions with a common denominator
Given fractions are:
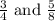
To make the denominator of both fractions common, we have to find L.C.M of denominators (Least common multiple )
Here the denominators are 4 and 8
L.C.M of 4 and 8
List all prime factors for each number.
Prime Factorization of 4 is: 2 x 2
Prime Factorization of 8 is: 2 x 2 x 2
For each prime factor, find where it occurs most often as a factor and write it that many times in a new list.
The new superset list is
2, 2, 2
Multiply these factors together to find the LCM.
LCM = 2 x 2 x 2 = 8
Thus L.C.M of 4 and 8 is 32
Given fraction is:
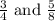
Multiply the denominator by a number so that it becomes 32 and multiply that same number with numerator also
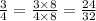
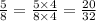
Thus the pair of fractions as a pair of fractions with a common denominator of 3/4 and 5/8 is