Answer:
Dimensions of each pen are
 and
and
 .
.
Explanation:
Please find the attachment.
We have been given that a rancher decides to make 4 identical and adjacent rectangular pens against her barn each with an area of
 .
.
The area of rectangle is width times length, so we can set an equation as:
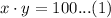
The fence of 4 identical and adjacent rectangular pens will be equal to perimeter of 4 adjacent rectangles as:
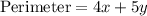
From equation (1), we will get:
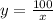
Upon substituting this value in perimeter equation, we will get:
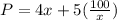
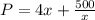
Now, we will find the first derivative of perimeter equation as:
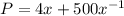
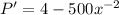
Now, we will equate 1st derivative equal to 0 to find the critical points:
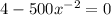
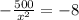
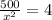
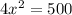
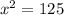
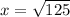

Now, we will find 2nd derivative of above equation as:
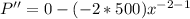

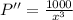
Now, we will check point
 in 2nd derivative, if it is positive, then x will be a minimum point.
in 2nd derivative, if it is positive, then x will be a minimum point.
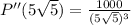

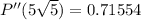
Since 2nd derivative is positive, so fence will be minimum at
 .
.
Now, we will substitute
 in equation
in equation
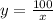 to solve for y as:
to solve for y as:
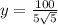
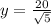

Therefore, the fence will be minimum at
 .
.